How to debunk evidence of a flat earth

A hobby of every Flat Earther is to show the flat earth through pictures of mountains. Of course, this can only go wrong, because the earth is almost a sphere.

But how to debunk this false evidence?

The most important thing is to find a reference for the sizes in the picture. Pictures with sunset are particularly suitable because the angle of the sun is about 0.5°. Since the images or videos are usually of poor quality, this level of accuracy is entirely sufficient. The sizes in the pictures can mostly only be determined approximately. The fluctuations in the distance between the sun and the earth are not so large that they affect the evidence.

Another way is to identify more mountains and determine the heights above the horizon.

I did that a few years ago with Tenerife (sun) [Ist die Erde flach? (Teil 3)] and Mallorca (mountains) [Ist die Erde flach? (Teil 6)]. Now I have the opportunity to demonstrate this on two recent videos.

Example 1 : Tête de l’Estrop

Let’s look at the first video at minute 2:11. Just Open Your Eyes … The Earth is FLAT (Old Taboo) from @tabooconspiracy.

At minute 2:11 the sun is behind the mountains. It is 205 pixels high and 252 pixels wide. So 0.5° corresponds to about 205 to 252 pixels. The tallest mountain is 47 pixels high, which roughly corresponds to an angle of 0.093 to 0.115°. You can’t measure it that precisely. We can’t be sure that this is really Tête de l’Estrop, but let us assume @tabooconspiracy is right and the picture was taken from Pic de les Nou Fonts. Or (Pic de Noufonts)

Mountain	Lat	Lon	Distance	Height	Angle	Horizon
Pic de Noufonts	42,42472	2,16750	407,2	2.861	0,403	191,0
Tête de l’Estrop	44,28722	6,50472	407,2	2.961	0,417	194,3

As describe in the video, mountain Tête de l’Estrop is 407.2 km away from Pic Noufonts. At an altitude of 2,961 m, the elevation angle above the horizon should be 0.417° on a flat earth. So it’s missing 72.5% to 77.6% or 2147 m to 2299 m hidden behind the horizon.

The horizon around Tête de l’Estrop is 194.3 km, the horizon around Pic de Noufonts is 191 km. The total is less than 407 km. Therefore, the Tête de l’Estrop should not be visible from the Pic de Noufonts. But we just wanted to prove that if it’s the Tête de l’Estrop in the picture, it’s not fully visible and the earth must be curved.

However, if the earth were flat, the mountain should be fully visible, which it is not. Case closed.

Our proof is successful. It doesn’t have to interest us why we see mountains there and which mountains they are.

What we can see and what we are likely to see are the mountains around Marseille.

Conclusion:

Perfect proof that the earth cannot be flat. Just open your eyes and do some math.

Exampel 2: Hawaii

Let’s look at the first video at minute 2:11. Hawaii proves FLAT EARTH from @flatearthclock.

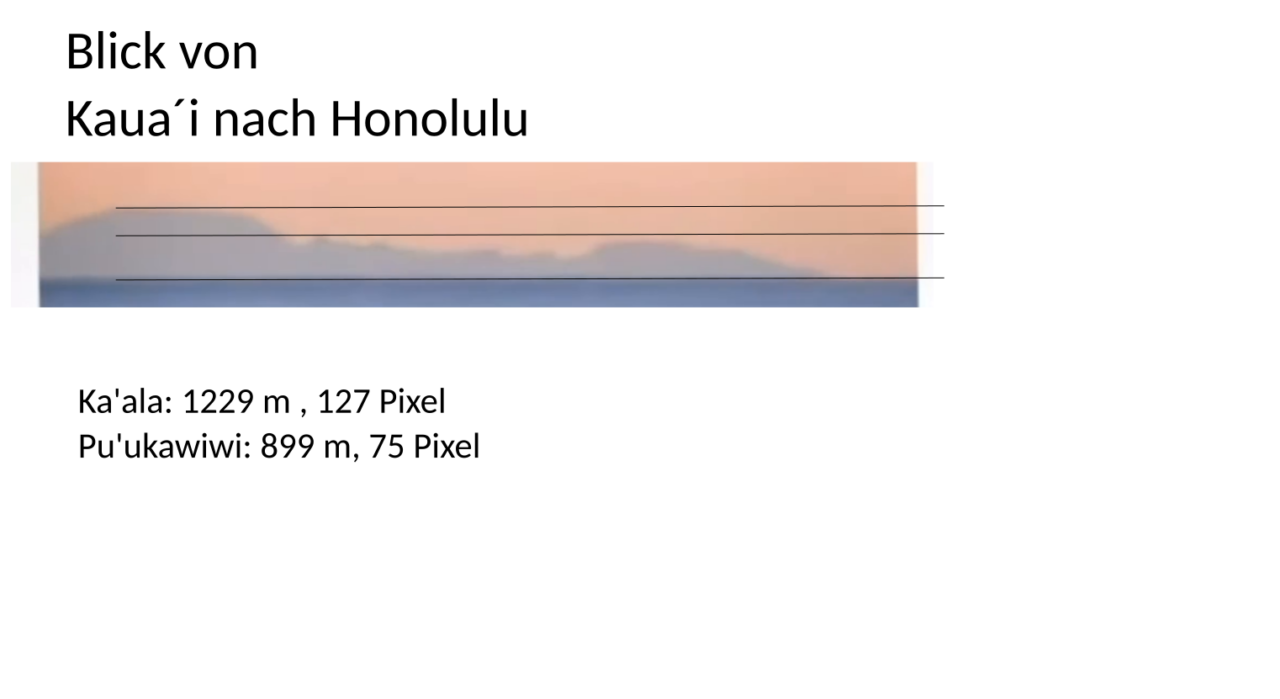
The picture shows Honolulu from Kaua’i. Exactly where it was taken from is not of interest to us at the moment. The highest mountain in Honolulu is Ka’ala at 1229. It is 127 pixels in the picture
high. Roughly to the right it first goes steeply downhill and then comes the Pu’ukawiwi with 899 m as the next higher mountain. Here in the picture, re is about 75 pixels high. That doesn’t look bad, but it is. At Ka’ala, 1 pixel corresponds to 9.7 m and at Pu’ukawiwi to 12 m. The following formula can be used to calculate the height h_h hidden behind the horizon:

h_h = (p₂h₁-p₁h₂)/(p₂-p₁)

Mountain	Height	Pixel	Meter/ Pixel	Hidden
Ka’ala:	1229	127	9,7	423,04
Pu’ukawiwi:	899	75	12,0

No matter where Honolulu was photographed from, about 423 m of the mountains are obscured by the horizon.

At 423 m eye level the horizon ist 73.4 km away. The distance between Kaua’i to Ka’ala is approximately 143 km. We need a viewpoint with an eye level of 385 m on Kaua’i to fill the gap of 70 km.

There is a Tree Tunnel lookout on Kaua’i that is near a busy road as seen in the video. However, this vantage point is not 200 ft high, but rather around 385 m. Which would fulfill the requirement.

But that doesn’t matter to us. Such an image can easily be taken on a terrestrial globe. So the picture doesn’t prove that the earth is flat, but it proves that the earth is curved at this point.

Conclusion:

Perfect proof that the earth cannot be flat. Just open your eyes and do some math.